We are given three shapes to test a half circle triangle and square total length of.
Calculus optimization worksheet a rain gutter is to be constructed.
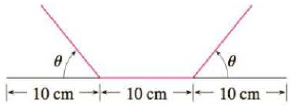
By bending up one third of the sheet on each side through an angle θ.
A rain gutter is to be constructed from a metal sheet calculus.
The problem asks me to find the best shape for a rain gutter in terms of how much volume will flow down it.
Here are the steps in the optimization problem solving process.
Verify if it is a maximum or minimum using the 2nd derivative test when easy otherwise use the 1st derivative test.
Calculus worksheet on optimization work the following on notebook paper.
Give all decimal answers correct to three decimal places.
Maximizing or minimizing some quantity so as to optimize some outcome calculus is the principal tool in finding the best solutions to these practical problems.
Differential calculus published in vacaville california usa a rain gutter is to be constructed from a metal sheet of width 30 cm.
Label the picture using variables for unknown quantities.
2 sketch a picture if possible.
Write a function for each problem and justify your answers.
Thanks to all of you who support me on patreon.
A rain gutter is to be constructed from a metal sheet of width 30cm by bending up one third of the sheet on each side by an angle theta from the horizontal theta zero represents the unbent sheet.
A rain gutter is to be constructed from a metal sheet of width 30 cm by bending up one third of the sheet on each side through an angle θ.
You da real mvps.
This tutorial teaches you to solve various calculus optimization examples and word problems that use derivatives and general differentiation techniques.
1 read the problem.
How should θ be chosen so that the gutter will carry the maximum amount of water.
Find two positive numbers such that their product is 192 and the sum of the first plus three times the second is a minimum.
Most real world problems are concerned with.
I am a first year calc student and am having a bit of trouble with an application problem.
1 draw a diagram depicting the problem scenario but show only the essentials.
How should θ be chosen so that the gutter will carry the maximum amount of water.
Optimization problems name calculus ab for each of the following define your variables write an equation representing the quantity to be maximized or minimized and solve the problem.
1 per month helps.
Determine what theta should be chosen so that the gutter will carry the most water when it is full.